S820E Microwave Site Master™ User Guide : Cable and Antenna Measurements : Trace
 
Trace
The Site Master S820E allows you to concurrently view the live trace plus a second trace that is stored in trace memory. You can compare the two traces visually or by using trace math functions. Pressing the Trace (5) main menu key brings up the trace functions.
Overview of Traces
• Recalled measurements (.dat files) from internal memory or a USB stick are automatically copied to trace memory and are then available to be displayed. To display the recalled measurement trace along with the live measurement, select Trace & Memory from the Trace Display menu.
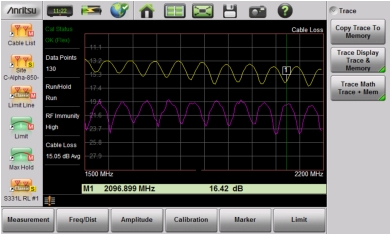
Note 
Recalled measurements may change the current instrument settings.
• Copy Trace to Memory replaces whatever is in memory with the live (yellow) trace. The memory trace (purple) is displayed behind the live (yellow) trace.
• The default view is live Trace Only. View options (Trace Display) also include viewing only the trace in memory or both traces.
• View only trace memory to have marker values apply to the purple trace.
Displaying a Live Trace and a Static Trace from Trace Memory
From the Trace menu you can Copy Trace to Display Memory. The copied trace can be displayed on the Site Master and used for trace math. Trace Display allows viewing of two traces at the same time to compare the trace stored in memory to the live trace. Trace Math operations include Trace ‑ Memory, Trace + Memory and (Trc + Memory) / 2. Saved traces can also be recalled and compared with the live trace.
Trace Overlay
The examples below illustrate how the trace overlay feature can be used to compare the return loss measurements between two cables.
1. Connect the first cable and setup the measurement. Refer to Measurement Setup for additional information.
1. Press Trace (5) and then Copy Trace To Memory.
2. Remove the first cable and connect the second cable.
3. Press Trace Display and select Trace & Memory. The purple trace from trace memory is displayed along with the live (yellow) trace.
Trace Overlay of Two Cables
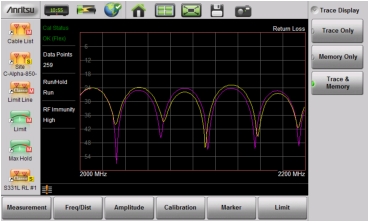Note 
The trace from memory can be displayed only if the measurement settings (except for Amplitude) have not changed since the trace was copied to memory.
If one of the traces is cut off, then pressing Amplitude > Fullscale adjusts the reference level to display both traces.
Trace Math Example
The example below illustrates how the trace math features can be used to compare the measurement of two cables.
1. Complete the steps described in Trace Overlay.
2. Press Trace Math and select Trace – Mem, Trace + Mem, or
(Trc + Mem) / 2 (Figure: Trace Memory Used to Compare Return Loss of 2 Cables).
.
Trace Memory Used to Compare Return Loss of 2 Cables
 
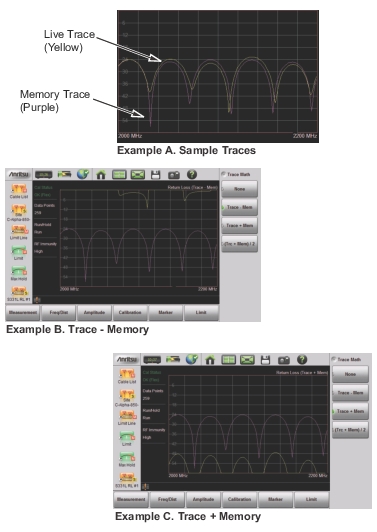Notes
The trace math functions often seem backwards to new users. The points to remember with Trace ‑ Memory,
Trace + Memory, and (Trc + Mem) / 2 are:
• The numbers on the y‑axis are negative.
• The purple trace is added to or subtracted from the live trace. The sum or difference of the live trace and memory trace is displayed in yellow.
 
Trace Math Details (Example from Figure: Trace Memory Used to Compare Return Loss of 2 Cables) (1 of 2)
Example
Example Description
A. Sample Traces
Shows the live yellow trace and purple memory trace.
B. Trace ‑ Memory
In the Trace – Memory graph, the yellow trace is the result of subtracting the purple memory trace from the active trace.
(The active trace is displayed in Example B, Trace – Memory as the yellow trace, and appears different because trace math is applied to it.)
Note that the yellow Trace – Memory is at 0 or above (and off the graph) whenever the yellow trace (as shown in Example A) is above (has a greater value than) the purple trace.
The two down sloping bumps in Example B are when the purple trace (in Example A) moves above the yellow trace. In Trace – Memory, this results in a negative value being displayed.
C. Trace + Memory
In the Trace + Memory graph, the yellow trace is the result of adding the purple trace to the active trace.
(The active trace is displayed in Example C, Trace + Memory as the yellow trace, and appears different because trace math is applied to it.)
Note that the yellow Trace + Memory is below 60 (and off the graph) whenever adding the yellow trace value to the purple trace value is greater than 60 (refer to Example A).
(Trace + Memory) / 2
(not shown)
In the (Trace + Memory) / 2 graph, the yellow trace is the result of adding the purple trace to the active trace and then dividing the result by 2.
This math function is most useful when measuring one‑port Cable Loss (using the Cable Loss measurement).
1. Connect a Short to the end of the cable and store the trace into memory.
2. Next, connect an Open to the end of the cable and apply (Trc + Mem) / 2 math function.
Because the ripple generated by the Short and Open are 180° out of phase, the effect of this math function will be to cancel out the ripple, resulting in a more accurate cable loss measurement.
Refer to Trace Menu for additional information.